Completing the Square (Quadratic Equation)
Definition

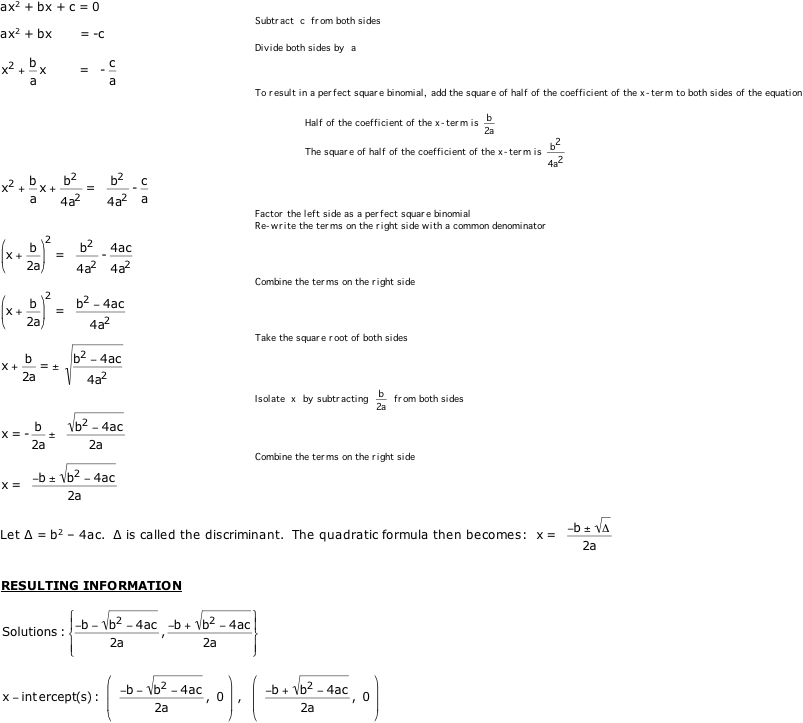
A process for solving a quadratic equation expressed in general form [ax2 + bx + c = 0]


Solving a quadratic equation is equivalent graphically to determining the x-intercept(s).

General Process

Problem

Solve   ax2 + bx + c = 0   by completing the square, then state the x-intercept(s) of the graph of the corresponding parabola.


Solution

Derivation of the Quadratic Formula

Example One

Problem

Determine the solutions of   y = x2 + 6x + 5   by completing the square, then state the x-intercept(s) of the graph of the corresponding parabola.


Solution

Example One - Solution

Click here to see the graph of   y = x2 + 6x + 5.


Example Two

Problem

Determine the solutions of   y = -3x2 + 12x - 9   by completing the square, then state the x-intercept(s) of the graph of the corresponding parabola.


Solution

Example Two - Solution

Click here to see the graph of   y = -3x2 + 12x - 9.


Example Three

Problem

Determine the solutions of   2x2 - 5x + 1 = 0   by completing the square, then state the x-intercept(s) of the graph of the corresponding parabola.


Solution

Example Three - Solution

Click here to see the graph of   y = 2x2 - 5x + 1.