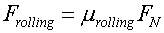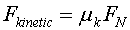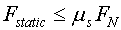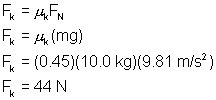Lesson 1 - Friction
Friction
simulates the force exerted on a spring (force) scale as it pulls a stack of
books across a rough table surface in order to explore the difference between
kinetic and static friction.
Prerequisites
Students will require a working knowledge of normal force, weight, free body
diagrams, and graphical analysis.
Learning Outcomes
Students will be able to graphically determine an expression for kinetic friction
and calculate kinetic, static and rolling friction in a variety of situations.
Students will also be able to qualitatively and quantitatively compare kinetic,
static, and rolling friction.
Instructions
Students should understand the applet functions that are described in Help and ShowMe.
The applet should be open. The step-by-step instructions on this page are to
be done in the applet. You may need to toggle back and forth between instructions
and applet if your screen space is limited.
Contents
- Comparing Kinetic and Static Friction
- Developing an Expression for Kinetic Friction
- Summarizing the Force of Friction
- Calculating Frictional Force - Examples
- Problem Solving
1. Comparing Kinetic and Static Friction
|
The applet will be used to demonstrate the difference between Kinetic
and Static Friction.
|
On the applet, stack the five books that are attached to the force scale
as shown below.
a. Using the mouse, slowly pull on the open ring of the force scale and
observe the reading on the scale just as the stack of books begins to
move.
What is the maximum force applied before the books start to
move? ________ N
b. Once the books begin to move, the force required to keep the books
moving is reduced.
What is the applied force required to keep the books moving?
_________ N |
Before the stack of books begins to move, the force of friction is called
Static Friction. After the motion begins, the stack of books is
subject to Kinetic Friction. In your own words, describe the relationship
between the size of the Static Frictional Force and the Kinetic Frictional
Force.
|
Click "FBD" ( )
to display the FBD drawing. In Figure 1, the red vector represents the
frictional force (Ff) while
the brown vector represents the applied force
(F). Note the behavior of the FBD as you pull on the force
scale.
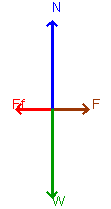
Figure 1
a. What happens to the size of the static frictional force as you start
to pull on the force scale?
b. Is there a maximum size for the static frictional force? If so, what
happens to the object if the applied force exceeds the maximum static
frictional force?
c. Is there a minimum size for the static frictional force? If so,
under what conditions will the minimum static frictional force be observed?
d. What type of frictional force acts when the system is at rest?
|
2. Developing an Expression for Kinetic Friction
The force of friction arises from an interaction between
surfaces. In the case of the books and rough table surface, the table surface exerts
a force on the bottom book. This force is the normal
force (FN)
and is represented in blue on Figure 1. Opposing the normal force is
the object's weight. The weight (W) is
represented in green on Figure 1. For the books resting on the table,
the normal force is equal in magnitude to the weight. The normal force
and weight are "balanced". The magnitude of the normal force
can be calculated by equating it to the weight. For example,
FN
= W
FN =
mg
Even though friction is an extremely complex phenomenon, there is a very simple
relationship between the magnitude of the frictional force and the normal force.
a. Using the applet, measure the applied force required to keep the books moving
and complete the following table. To calculate the normal force, assume that
each book has a mass of 1.00 kg.
b. Complete Graph 1 by plotting the Normal Force on the x-axis (manipulated
variable) and the Kinetic Frictional Force on the y-axis (responding variable).
| Number of Books |
Normal Force (N)
FN = mg |
Kinetic Frictional Force (N) |
| 1 |
_________ |
_________ |
| 2 |
_________ |
_________ |
| 3 |
_________ |
_________ |
| 4 |
_________ |
_________ |
| 5 |
_________ |
_________ |
| 6 |
_________ |
_________ |
| 7 |
_________ |
_________ |
| 8 |
_________ |
_________ |
| 9 |
_________ |
_________ |
| 10 |
_________ |
_________ |
Kinetic Frictional Force vs.Normal Force
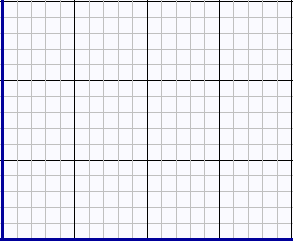
Which of the following
best describes Graph 1: Kinetic Frictional Force - Normal Force? Use
your answer to write an equation expressing the relationship between Kinetic
Frictional Force and the Normal Force.
-
The graph is constant and of the mathematical form y = b (b
is constant).
-
The graph is linear and of the mathematical form y = mx + b (b
is zero and m is the slope).
-
The graph is a quadratic curve and of the form y = ax2 +
bx + c (a, b and c are coefficients).
Equation: ________________
The equation should
have the form y = mx where m is slope of the equation. This
means that the equation for the kinetic frictional force is Fkinetic
= μFN where,
| Fkinetic
= |
kinetic force of friction (y-axis) |
|
FN = |
normal force (x-axis) |
|
μ= |
coefficient of kinetic friction
(slope) |
Using Graph 1, calculate the coefficient of kinetic friction (μ) for the
book - table interface. According to the slope calculations, does μ have
any units?
3. Summarizing the Force of Friction
Frictional forces are complicated. They depend not only on the two objects involved
but also their conditioning at the moment of the interaction of the two objects.
For example, roads made of asphalt become very slippery when freezing rain or
snow covers the surface and tires lose their grip when they are worn. Furthermore,
even if all these circumstances are fixed, the frictional force also depends on
whether an object rolls, slides (kinetic), or is stationary (static) with respect
to the other surface.
This gives rise to three basic forms of friction:
Rolling Friction
|
Kinetic Friction
|
Static Friction
|
|
A rolling tire will still experience a small frictional
force.
|
When an object slides, it experiences a frictional
force.
|
When an object is at rest a static frictional force
resists motion.
|
|
|
|
|
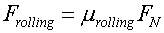
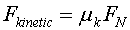
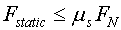
Usually, rolling frictional forces are much smaller
than kinetic and static frictional forces.
|
Kinetic frictional force always opposes motion.
It acts opposite the direction of motion.
|
The maximum static frictional forces are larger
than the kinetic frictional forces.
|
* μrolling, μk and
μs are the coefficient of rolling, kinetic, and static
friction and FN is the normal force.
4. Calculating Frictional Force - Examples
1. The coefficient of kinetic friction between a block
and the level surface it slides on is 0.45. If the mass of the block is
10.0 kg, what is the minimum force needed to keep the block moving
with uniform motion?
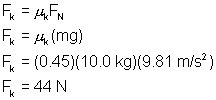
The applied force need only balance the kinetic frictional force in order
to maintain uniform motion.
|
2. A student pulls on a 5.00 kg object and discovers
that she needs to exert 30.0 N of force before the object moves.
What is the coefficient of static friction between the object and the
surface on which it rests?
Since the applied force is 30.0 N just before moving, the
frictional force must be equal in size. Therefore,
|
3. A 7.30 kg box is at rest on a level table. The
coefficient of static friction between the box and table is 1.03. How
big is the static frictional force?
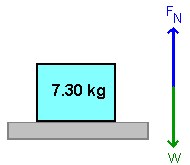
There is no static frictional force acting since there is no net force
attempting to move the box . The static frictional force could, at most,
be equal to ustaticFN.
|
5. Problem Solving
 Devise
a method to determine the coefficient of static friction used in
the applet. Explain how you found the coefficient and give its value.
|
A student drew the free body diagram for a box sitting at rest on the
floor. Explain what is wrong with this diagram.
|
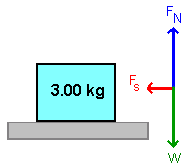
 The
coefficient of static friction between a book and the level surface
it slides on is 0.65. If the mass of the book is 2.0 kg, what is
the minimum applied force required to move the books?
 An engine
provides 300.0 N of force to keep a 1600 kg vehicle moving at
a uniform speed (air resistance is negligible). What is
the coefficient of rolling friction between the tires and the
road surface?
 A 100.0 kg
crate is at rest in the back of a truck. If the coefficient of
static friction between the crate and the truck bed is 0.30, what
is the maximum acceleration that the truck can have before the
crate begins to slide? Draw a Free Body Diagram and explain
what force causes the crate to accelerate along with the truck.
Anti-lock brakes prevent car tires from locking up and skidding. Explain
why a car can stop faster and safer if the tires do not skid.
|
Physics 20-30 v1.0
©2004 Alberta Learning (www.learnalberta.ca)
Last Updated: June 16, 2004