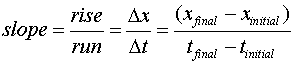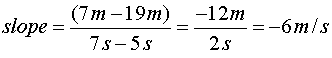Lesson 1 - 1D Uniform Motion Builder Graphing (pos, vel)

Example: A student is jogging along a straight path. The foloowing graph shows her position (measured from her starting position) plotted against time.

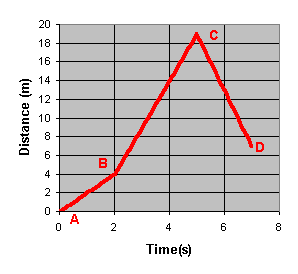
1. Answer the following questions.
   
   1. What is the greatest distance she traveled from her starting point, and when did this occur?
      
      (Answer: By inspection of the graph, the highest value for the distance is 19 m and this occurs at t = 5 s.)
      
      
   2. What is different about her motion from 0 - 5 s (points A - C) compared to her motion from 5 - 7 s (C -D)?
      
      (Answer: From A - C the distance from the starting point is getting bigger. After this point, the distance begins to decrease. She must have turned around and is now running back.)
      
      
   3. For what part of her motion was she traveling the fastest? Explain your answer.
      
      (Answer: Between point C - D she traveled (19 m - 7 m) = 12 m in a total of (7 s - 5 s ) = 2 s. Her rate of speed was (12 m)/(2 s ) = 6 m/s. This is faster than any other section of the graph.)
      
      

2. Eager to get to Physics class, a student pulls out of the driveway and "floors-it"! The following graph shows the position of his car as a function of time.

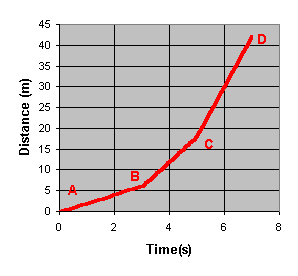
1. How far did the car travel in the first 5 seconds?
   
   
   
   
2. When did the car have its highest speed - explain how you can tell this just by looking at the graph (no calculations).
   
   
   
   
3. Calculate the highest speed that the car had over the seven seconds plotted here.
   
   
   
   
   
4. What was the average speed of the car over the 7 second interval? (Remember, average speed is defined by:)
   

3. The following graph shows the velocity -time graph for a radio controlled race car.

1. What is the fastest speed for the model car? When does this occur?
   
   
   
2. What is the lowest speed (careful - there are two answers!) and when does this occur?
   
   
   
3. Can you tell how far the car traveled just by looking at the graph?
   
   
   
   
4. The graph shows four distinct "phases" for the motion. Fill out the following table and then enter these values into the applet1D Uniform Motion Builder Graphing (pos, vel) and reproduce the graph shown above.
   
   

   Time Interval (s)	Velocity (m/s)
   0 - 1	______
   1 - 2	______
   2 - 3	______
   3 - 7	______

4. The velocity-time graph for an object is shown below.

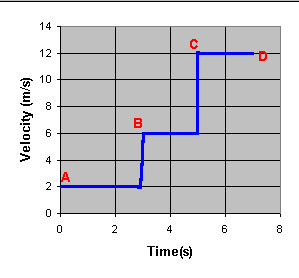
1. How fast was the object moving between t = 3 seconds and t = 5 seconds? How far did it travel during this interval?
   
   
   
2. How could you determine the distance that the object would travel for the entire 7 seconds plotted here?
   
   
   
   
   
3. There are three distinct phases for the motion plotted here. Identify these phases and fill out the following table. Use this information and the applet 1D Uniform Motion Builder Graphing (pos, vel) to reproduce the graph shown here.

The slope of a graph is a measure of how steep the graph is. We define slope mathematically as the ratio "rise/run". Rise on a Position-Time graph represents distance traveled and run represents the time taken. If we apply the "rise/run" idea here we get an expression for average velocity . The following graph is the same one that you saw in the example above.

We can write the following formula:

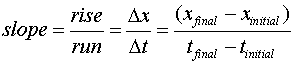
To see how this works, let's put in the correct numbers for x and t at the points "C" and "D". We then get:

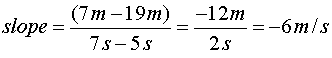
This is the velocity of the jogger in the example above for the interval C-D.

1. Find the velocity of the jogger from the example above for the intervals A-B and B-C shown on the previous graph.

2. The applet 1D Uniform Motion Builder Graphing (pos, vel) has the ability to show you slopes of position-time graphs. To see how this is done, enter the following time and velocity motion scripts in the applet:
   • script1: t = 2 s , v = 2 m/s
   • script2: t = 3 s , v = 5 m/s
   • script3: t = 2 s , v = -6 m/s

   Your graph should look like the one shown here.

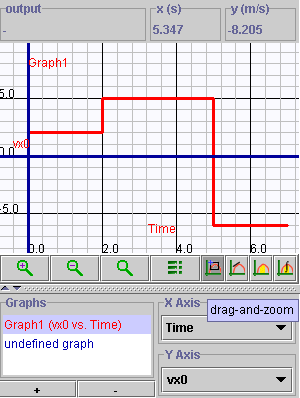
3. Use the slope tool to measure the slope for the three intervals that you plotted and verify that the slopes agree with the velocities that you entered.

4. In the space provided, sketch the velocity-time following graph for the motion you defined in question 6. Verify that your sketch is correct by using 1D Uniform Motion Builder Graphing (pos, vel).

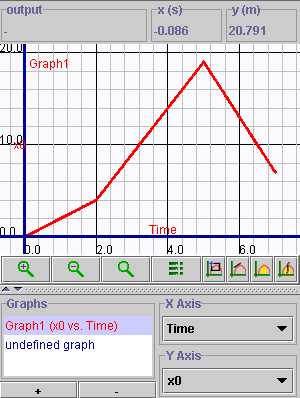
The following graph is very similar to the one you constructed in question 2b.

The graph agrees with the motion scripts that you entered and shows that:

• between t = 0 s and t = 2 s the jogger moved with a velocity of 2 m/s
• between t = 2 s and t = 5 s the jogger moved with a velocity of 5 m/s
• between t = 5 s and t = 7 s the jogger moved with a velocity of - 6 m/s
  
  

1. How far did the jogger travel in the first 2 seconds? How far did the jogger travel between t = 1s and t = 4 s?

The distance calculations that you did in question 8 were very easy and you had no trouble showing that the distances traveled were 4 m and 12  m respectively. You should also be able to see that these numbers are exactly the area between the velocity-time graph and the time axis. The following statement reflects a very important property of all velocity-time graphs.

The area between a velocity-time graph and the time-axis for a given interval of time is equal to the displacement of the object in that interval of time.

You can use the graphing features of the 1D Uniform Motion Builder Graphing (pos, vel) applet to easily verify this. Select the area tool and show that the distance traveled between t = 1 s and t = 4 s is 12 m. Your graph should look like the one shown. Note the value displayed in the "output window".

2. Now consider the area for the entire interval 0 - 7 s. How far was the jogger from her starting point at t = 7 s? Why is this less than her position at t = 5 s?
   

3. It's important to know how to define your terms! Explain the difference between the terms 'distance' and 'displacement' as they are used in Physics.

4. Explain why the following statement, in general, is false: "The area between a velocity-time graph and the time-axis equals the distance traveled."

1. Melanie is sprint-cycling and gets off to a good start pedaling at a rate of 12 m/s for 10 s. She tires and slows down to 9.8 m/s for the next 16 s. When she gets close to the finish line she begins pedaling at 11.5 m/s for the next 2 s. Sketch her position-time graph and velocity-time graphs in the space below.
   
   
   
   
   
   
   
   

1. How far did Melanie travel?
   
   
   
2. What was her average velocity for the trip?
   
   
   
3. You may have learned to average numbers by "adding them up and dividing". Try that strategy here. Why does the answer you got in part b not agree with this? Which is correct?

2. Consider an object that speeds up by 10 m/s for every second that it moves. For example, during the first second it travels at 10 m/s, during the second second it travels at 20 m/s, and so on. Complete the following table:

1. In the space below sketch both the position-time and velocity-time graphs for this motion. (Try to do this without using 1D Uniform Motion Builder Graphing (pos, vel) and then check your results with the applet).
   
   
   
   
   
   
   
   
2. How would you describe the "shape" of the position-time graph? What familiar "curve" does it remind you of?
   
   
   
   
3. How would you describe the "shape" of the velocity-time graph? What would happen to the shape of this graph if you defined smaller and more numerous time intervals?
   
   
   
   
   
4. What very common kind of motion does the above example approximate?